(Status: This may be a relatively well-known topic, but apart from a single footnote I haven't found it mentioned here. For now I would rate my numeracy below the average person I'd expect to read this, so I expect there may be connections or applications left out that would be obvious to a graduate-level audience. For a drier, more rigorous introduction, I'd recommend the Aljefri (2018) paper in the citations.)

Hypergames

Hypergames are game-theoretic objects that model information asymmetry and misperception between players. Each player has a separate payoff matrix, and their preferences, available choices, and even awareness of the number of other players may be different. Because of this, hypergames have been used in the literature to model international conflicts, and to a lesser degree coordination problems.

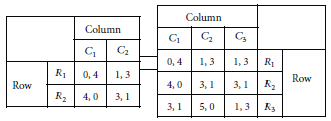
A chief feature of a hypergame is that to any individual player it can look and behave just like a classical game, so a player can be playing a hypergame without knowing. It's equally possible, however, to be aware that you're in one and try to model the perceptions of other players before making any decisions yourself. This can be described as trying to determine another player's rank-ordering of the choices available, or their "preference vector", and can lead recursively to agents predicting each other's predictions, given as N-level predictions of (N-1)-level predictions. 

Sometimes a hypergame is named after the highest level of prediction in it, making it an "N-level hypergame". If at least one player is aware that a hypergame is happening, then the game itself must be at least 2nd-level. 

Hypergames can be reduced into classical games if perfect information is achieved - all the payoffs and choices are simply collated into one matrix and the classical tools can be brought to bear. In cases of conflict this is much harder to achieve, but because of this equilibria are classed as either "hypergame-preserving" or "hypergame-destroying" based on whether all players perceive them as equilibria or some gain unexpected information from them. 

One motivation for studying hypergames in particular is stability analysis, the study of decisions in a game that an agent wouldn't opt to change. Some strategies are stable for the set of options a player perceives but unstable in the game-in-full, and it's possible for a stable strategy to exist in reality that requires personally-unstable choices on the part of every player involved. 

From here my ability to explain is limited, and I'd recommend looking into the papers below, as there seems to be relatively little in the literature on this subject and much of it is combined with other advances like including dynamic variables or fuzzy logic in payoff matrices. 

Citations

Keith W. Hipel, Muhong Wang & Niall M. Fraser (1988): Hypergame Analysis of the Falkland/Malvinas Conflict, International Studies Quarterly, 32(3), 335-358.

Nicholas S. Kovach, Alan S. Gibson & Gary B. Lamont (2015): Hypergame Theory: A Model for Conflict, Misperception and Deception, Game Theory, 'Vol 2015'.

Yasir M. Aljefri, Keith W. Hipel & Liping Fang (2018): General hypergame analysis within the graph model for conflict resolution, International Journal of Systems Science: Operations & Logistics.

Yasir M. Aljefri, M. Abul Bashar, Liping Fang & Keith W. Hipel (2017): First Level Hypergame for Investigating Misperception in Conflicts, IEEE Transactions on Systems, Man, and Cybernetics: Systems, Vol 48.