Note: This is the first post in a sequence I announced over a year ago. The sequence is intended to serve as a primer to the field of economic growth for those interested in the intersection between growth theory and artificial intelligence. See the announcement for more details.

Economic growth theory concerns the long-run behavior of economies, such as the United States, or the world economy. An "economy" here simply means the collective behavior of many agents interacting in a universe with scarce resources. Since economies are very complex, economists tend to focus on a small set of summary statistics to characterize them. For our purposes, we are most interested in the behavior of gross domestic product (GDP) over time as it interacts with factors such as labor, capital, and technology. The Solow-Swan model is the benchmark model in mainstream economics for understanding this process, so it is the first model we will look at in this sequence.

Why GDP?

Technically speaking, GDP is the market value of all final goods and services produced within a given nation, in one year. The meaning of "final good" is crucial here: if someone is producing a good for an intermediate purpose—such as smelting metal to be used in a smartphone—only the value added to the smartphone is counted for the purpose of GDP, not the total money exchanged during the entire process of making the smartphone. This concept is crucial for understanding the so-called production approach to calculating GDP.

GDP is useful for characterizing a large economy because, in two senses, it represents both the output and input to a market economy. GDP represents the output of an economy for a reason I have already alluded to: it is estimated by counting up all production within the economy, with only those interactions between agents that add value to end consumers counting in the calculation.

GDP also represents the input to a market economy because each good produced will at some point be bought by a consumer. There are two approaches to calculate GDP which take this fact into account. The first is the expenditure approach, which adds up the market value of all goods and services sold to consumers within an economy over a year. The second is the income approach, which is based on the fact that each good and service bought by a consumer also represents income taken home by a seller.

From the perspective of development economics, GDP is a critical statistic because it is highly correlated with, and likely determines, the standards of living within a nation. For example, Jones and Klenow (2016) devised an expanded welfare metric taking into account "consumption, leisure, mortality, and inequality" and found a high correlation between per capita GDP and their welfare metric.

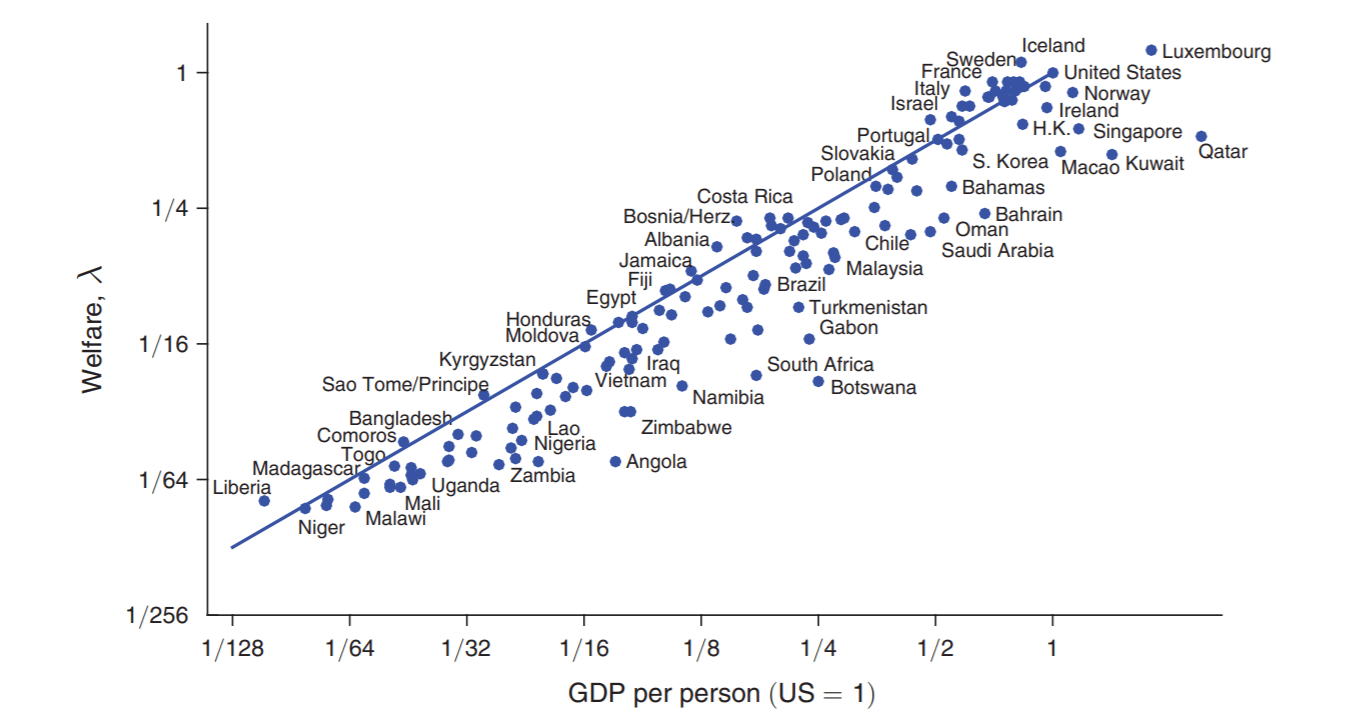
GDP per capita is also highly correlated with nutrition, reported life satisfaction, absence of disease and disability, among many other measures of human welfare.

It would be no surprise if the relationship between GDP and human welfare is directly causal. After all, the income approach to calculating GDP shows that all GDP should represent someone's income. Therefore, the more GDP in a nation, the richer that nation is in aggregate terms, which implies that people have more money to spend on goods and services that can improve their life.

From the perspective of artificial intelligence, GDP can arguably be used to measure the impact of AI technologies, at least in the medium-term. The reason comes from treating AI as a product used in the production of goods and services, sold in a market economy.

For at least the last few centuries, engineers have developed tools that enable us to produce goods and services more efficiently than before, requiring less human labor for the same real (ie. inflation adjusted) output. This process of automating tasks is at the heart of why standards of living have risen so dramatically in such a short period of time. A task that used to take 100 workers 10 hours to complete, now might take only 10 workers 1 hour to complete, freeing up labor for other productive uses.

The most obvious use-case for AI is to continue this trend of rising productivity, enabling extremely high levels of production and consumption. As we shall see, studying the dynamics of GDP over time can give us insight into what role AI may play in the world economy in the future.

Production functions

Before I get to the meat of this post, the Solow-Swan model, I must first discuss so-called aggregate production functions. The reason is that the Solow-Swan model is really two models glued together; the first part being a production function, and the second part being a differential equation which describes how an input to this production function (namely, capital) changes with time.

A production function is almost exactly what it sounds like. It describes the amount of output that an economy can produce sustainably, given a set of inputs. For most purposes—except when talking about business cycles—we assume that an economy will employ its resources efficiently and at full capacity; thus, the level of output that can be produced, will be produced.

The inputs to a production function typically include (at least) capital, labor, and technology. For simplicity, we'll first consider an economy with a single input: capital. As we will see, the essential form of the Solow-Swan model does not change very much when we introduce other factors of production.

As a side note, the word "capital" does not narrowly refer to financial capital, but rather the set of tools, machines, and infrastructure used in the production of goods. For example, a road is capital, because although it's not very valuable in its own right, it's useful for transporting other resources.

The single-variable production function takes the form

where $Y$ is the level of output, measured in GDP, and $K$ is the total amount of capital in the economy. There's an interesting rabbit hole you can go down investigating the unit of measurement for capital, but for the sake of simplicity, imagine $K$ represents a broad index that can be used to measure the total stuff available to workers for producing goods and services.

The most important fact to understand about this production function is that output experiences diminishing marginal returns with respect to $K$. This property makes sense when you imagine ordinary capital, like a shovel. If five workers are digging a hole, and they only have one shovel, giving them one more shovel might double their productivity. However, if those workers already have five shovels, giving them one more shovel might only add to their productivity if one of their current shovels is broken.

The idea of diminishing returns is captured mathematically by the following conditions, which establish concavity of the production function,

We also impose what are called the Inada conditions,

On their own, these conditions are quite weak. However, when paired with an equation for the evolution of capital over time, we will begin to see the first interesting applications of the Solow-Swan model.

Savings and capital depreciation

There are roughly two ways a person can use their income: spend it on consumption, or save it (such as in a savings account, or a stock brokerage). 

Individually these actions are quite different; the first involves losing some money and getting some immediate utility, while the other involves holding onto money in the expectation of future utility. Yet, from the perspective of the economist, both of these actions technically represent spending, only differing in what money is spent where.

To be more specific, when an individual saves money, such as with a bank, the bank uses that money to make loans to other individuals, who go on to spend it. One supporting assumption of the Solow-Swan model is that all money saved in the economy is ultimately spent on capital investments. In fact, we define aggregate savings to be the same as the aggregate spending on capital goods.

Now consider that in addition to capital accumulating from investment, capital also depreciates from wear and tear over time. Furthermore, let's assume that some constant fraction $\delta$ of the capital stock depreciates per year, no matter the value of $K$. Then, we can represent the net investment in capital during some year as

where $s$ is the national savings rate, and $\delta$ is the capital depreciation rate. In a continuous setting, the evolution of capital over time is given by this differential equation:

The equation above is the central equation underpinning the Solow-Swan model, as it reveals the time path of capital given some initial capital stock. Furthermore, since output is determined by capital, this equation also describes the time path of output. From there, we can calculate the rate of economic growth, $\frac{d}{dt}Y=\frac{d}{dt}F\left(K\right)$.

To see how we can extract useful information from the equation of motion for capital, remember that we assumed that capital experiences diminishing returns, while depreciation remains constant no matter the capital stock. Graphically we can show this relationship with Figure 1.1 in Philippe Aghion and Peter Howitt's The Economics of Growth,

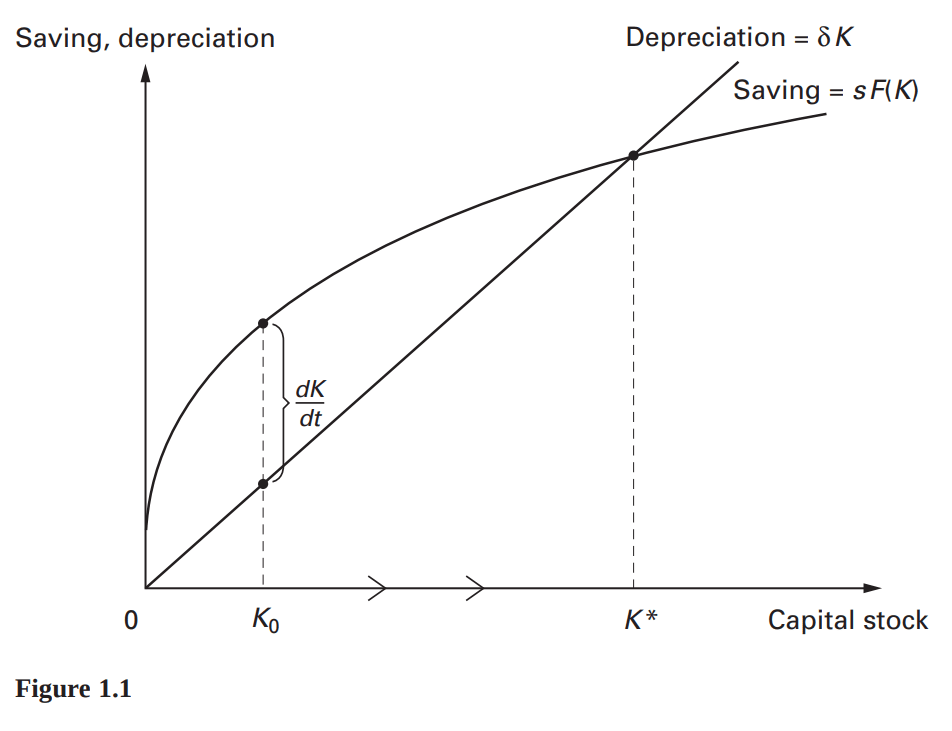
Observe that when the capital stock is small (but not too small), the difference between savings and capital depreciation is large. Since savings minus depreciation determines the rate of growth of capital, and capital determines output, economies will a small capital stock will grow quickly. However, as the capital stock gets larger, the difference between savings and capital depreciation gets smaller, implying that growth will slow down over time. This behavior is analogous to the phenomenon of poor nations growing quickly and rich nations growing slowly.

This slowdown in growth as a nation gets richer is ultimately just a reflection of the diminishing returns to capital. Think of it this way: if a government spends a large sum on building roads, eventually the economic benefits from having more roads will match the cost of repairing the roads that already exist, and marginal investments won't work to increase output anymore.

The equilibrium point $K^{\ast }$ represents the amount of capital that this economy tends to over time, given some fixed savings and capital depreciation rate. In other words, assuming $s$ and $\delta$ remain constant, and we start with some initial capital stock $K_0>0$, then ${lim}_{t\to \infty }K=K^{\ast }$.

This conclusion is said to be "pessimistic" because of its implication that, in the long run, sustained economic growth is not possible. Eventually, the economy will expand to the point at which new investments in capital simply make up for the depreciation of old capital, resulting in zero net economic growth; we'd have eternal stagnation.

However, from another perspective, the Solow-Swan model provides an optimistic view of growth policy. To see why, suppose we increase the savings rate of the economy. In that case, the savings rate curve in Figure 1.1 will be scaled upwards and a new stationary point $K^{\ast }$ will emerge at a higher level than before. As a result, the total output of the economy will also increase.

Yet, the policy lesson to be learned here is not simply "increase the savings rate as much as possible if you want maximum economic growth." That would be true if we only wanted as much output as possible. But people don't just want maximum economic output, if doing so means they can never spend money on consumption; always saving for the future, never living for the now. What we really want is the Golden Rule savings rate, defined as the level of savings such that total growth in consumption over time is maximized (see link for derivation).

Population growth and technology

As I stated earlier, capital is not the only input to production within an economy. Population growth, along with better technology, contribute to economic growth. The good news is that accommodating these inputs to the Solow-Swan model will only require a slight extension of ideas we have already covered.

To incorporate labor, we write the production function as

We also assume, as before, that this production function experiences diminishing returns to its inputs, characterized by the conditions for concavity in a two dimensional setting,

Don't worry if these equations don't immediately mean anything to you. What's important to recognize is that they simply mimic the single-variable case of diminishing returns. In other words, we can assume positive but diminishing returns with respect to any variable.

We incorporate technology into this model by assuming there is some technology level $A$ which acts to multiply total production given the capital and labor inputs. In other words,

The most popular production function satisfying these conditions is the "Cobb-Douglas production function", written

The exponents $\alpha$ and $\beta$ together determine the returns to scale of the labor and capital inputs.

Decreasing returns to scale imply that if you scale $L$ and $K$ by some constant $\lambda$, the output will scale by some amount less than $\lambda$. We see decreasing returns to scale when $\alpha +\beta <1$. Increasing returns to scale, naturally, means the opposite: simultaneously scaling $L$ and $K$ by $\lambda$ produces an increase to the output greater than $\lambda$, and we see this when $\alpha +\beta >1$.

Most commonly, we will assume constant returns to scale. In other words,

Formally, this means $F$ is homogeneous of degree one in both arguments.

Why might we believe in constant returns to scale? Consider two neighboring islands, A and B. On island A, there exists a nation with 10 workers and 10 units of capital producing 100 units of output. However, island B is uninhabited, despite sharing the same underlying geography and natural resources as island A. If we copied everything from island A, including both the workers and the capital they use, and put it on island B, then we might assume that the total output between the two simply doubles. This is another way of saying that capital and labor exhibit constant returns to scale.

Alternatively, if the workers on each island increase their productivity by conducing scientific research, the output between the two islands might increase by more than two, as there will be twice as many researchers contributing to a shared body of scientific knowledge. Or maybe output will increase by less than two if the conditions on island B are not very hospitable to life.

The point I'm trying to make is that a lot about economic growth hinges on returns to scale. This is particularly important in the context of this sequence, considering the proposed mechanisms by which AI could accelerate growth, a topic I will return to eventually.

Long-term growth in per capita output

When I first introduced the Solow-Swan model of a single input, I noted its pessimistic conclusion that long-term economic growth is impossible. In fact, the situation is not so bad if we assume that labor and technology can grow forever.

Permanent population growth trivially implies permanent growth in output, since $\frac{\partial }{\partial L}F(L,K)>0$ for all $L$. However, if the population is growing, but the capital stock remains the same size, then intuitively the capital stock will become more diluted as more workers are sharing the same capital stock; thus, per capita output will decline.

Yet, per capita output does not necessarily decline with population growth, because new workers can also add to the capital stock. Given constant returns to scale ($\alpha +\beta =1)$, and assuming full employment ($\text{total population}=L$), per capita output is given in the Cobb-Douglass production function by

$K/L$ represents the per capita capital, which we denote $k$. We can then write the per capita production function as

Let's first consider the case where the level of technology stays constant, $A=1$. Assume that population grows at a constant exponential rate $n$, ie. $\frac{d}{dt}L=nL$. Since each additional person in the population causes $k$ to fall by $nk$, as capital becomes "diluted" by the extra person, the capital stock will evolve according to the equation

(For the full derivation, view the footnote on page 26 in section 1.2.1 of Philippe Aghion and Peter Howitt's The Economics of Growth)

This new equation for the evolution in per capita capital looks very similar to the growth in capital given in the single variable Solow-Swan model. So naturally, we get essentially the same pessimistic result from earlier, except in regards to per capita output rather than total output. To summarize, given a fixed savings rate $s$, the per capita capital stock will tend towards $k^{\ast }$, resulting in no new economic growth in the long run.

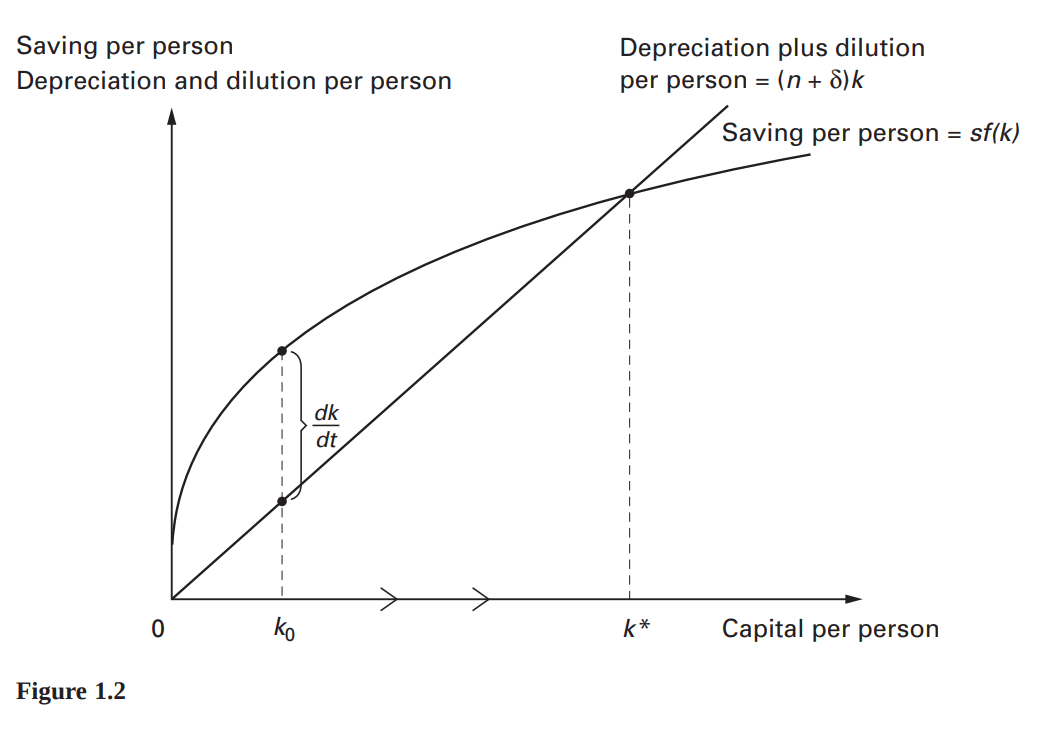
From this we conclude that in order to maintain permanent per capita growth in output, we must somehow maintain permanent technological progress. Labor and capital alone are insufficient; we need to figure out how to innovate.

But how do we get technological progress?

A while ago, I pulled out this parameter $A$ which was supposed to represent the technological level, but I didn't say much else about it, other than it scales the production function, yielding greater output for the same labor and capital inputs.

To someone knowledgable about scientific progress in the last few hundred years, it may have come as a surprise that the Solow-Swan model—the benchmark model in growth economics—has little to say about technological progress. Since progress in technology has driven the large majority of the rise in standards of living worldwide, the Solow-Swan model sheds little light on the last few hundred years of growth.

Yet the utility of the the Solow-Swan model can be seen in its account of non-technological related growth. To see why that might be useful, consider that labor and capital inputs are relatively easy to measure—governments do it all the time. Assuming the Solow-Swan model is correct, we can estimate what we'd expect economic growth to be given our labor and capital measurements alone. Comparing our estimate with the actual growth rate provides a neat way of measuring technological progress (the Solow residual).

This strategy is known as growth accounting, and it's a very general way of measuring hard-to-observe variables. For example, Danny Hernandez and Tom B. Brown arguably used a similar trick for measuring algorithmic progress in their paper for Open AI—while algorithmic progress is hard to measure on its own, their insight was that it can be inferred by first accounting for computational progress.

Ultimately, however, we still want a way of modeling technological progress, which the Solow-Swan model does not provide. Fortunately, the field of endogenous growth theory aims to provide a model of technological progress. The downside is that endogenous growth theory suffers from numerous methodological issues, stemming from highly sensitive initial conditions, unfalsifiability, and so-called "knife edge assumptions." Endogenous growth theory will be the topic of the next post.