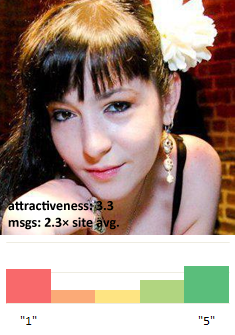
How subjective is attractiveness?
11Pablo
6Jacob Falkovich
4JonahS
1someonewrongonthenet
0JonahS
6someonewrongonthenet
2gjm
-7G-Max
8Nornagest
-7G-Max
4Nornagest
-5G-Max
7Kindly
0G-Max
6Jiro
0Kindly
7Nornagest
-7G-Max
5CCC
1Nornagest
0Wes_W
0Aiyen
-1G-Max
1Vaniver
-8G-Max
0CCC
0Aiyen
6CronoDAS
2JonahS
3kpreid
0JonahS
3Tenoke
3JonahS
5gjm
-6G-Max
6Richard_Kennaway
6[anonymous]
1Kawoomba
New Comment
Let me be an Excel sidekick among statistical analysis heroes.
I saw the OKCupid stuff as well, I ran a quick test in Excel to see if the variance in attractiveness contributes to the decision to meet beyond the attractiveness mean. Here's what I got doing regression, with apologies for the hideous formatting:
......... Coefficients ..Standard Error ..t Stat ..P-value
Intercept -0.569931558 0.042946471 -13.27074239 4.65749E-35
avg_attr 0.156634411 0.005238302 29.90175402 2.6299E-117
attr_std 0.028596624 0.012485497 2.290387431 0.022377128
The dependent variable is match percent (percent of people who decided they want to date the ratee), avg attr is the mean and attr std the standard deviation of the physical attractiveness ratings. attr std is not the attractiveness to STDs ;-)
As we can see, the coefficient for attractiveness deviation is significantishly positive. It actually has a small negative correlation with match and a larger negative correlation with attractiveness. This means that there is more consensus on the attractiveness of prettier people. Holding attractiveness constant, variance, which is visible for a single rater as an "unusual look", increases the chances that people will want to date you. Put some flowers in your hair!
Thanks :-).
I haven't looked at how the shapes of the distributions vary yet. The variability in standard deviations seems consistent with the phenomenon described in the OkCupid blog post, but I don't whether the high variance distributions tend to be bell curve shaped with larger standard deviations or bimodal.
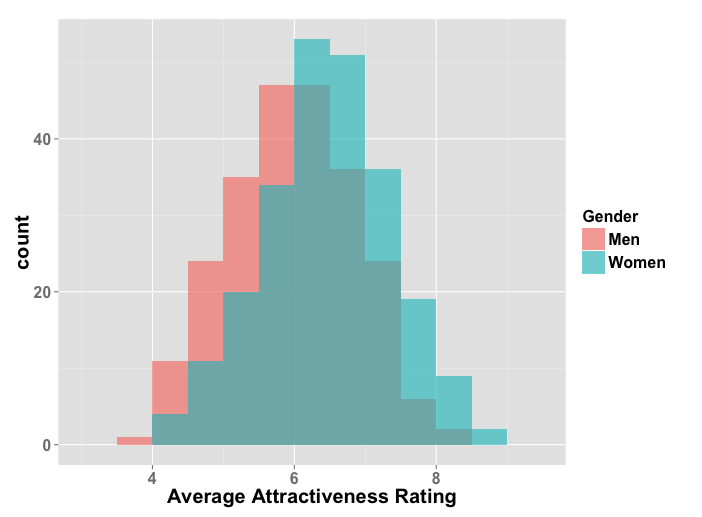
What is true is that there was no statistically significant secondary dimension of attractiveness. One would find other dimensions if there were a sufficiently large number of people at the events, but it's unclear how large "sufficiently large" is – it could be 10 more people, or it could be 100 more people. I'll be writing more about this later.
Undoubtedly, the homogeneity of the population studied also plays a role: if a woman with this facial adornment were at the event, and the event included some men from her culture, perceptions of her attractiveness would be extremely polarized.
Undoubtedly, the homogeneity of the population studied also plays a role: if a woman with this facial adornment were at the event, and the event included some men from her culture, perceptions of her attractiveness would be extremely polarized.
I hear this a lot, and the Mursi always used as an example.
I don't think attraction is that malleable. Personally, I suspect that as a culture the Mursi simply don't prioritize beauty. They have marriages which are arranged as children, with cattle as a medium of exchange. They probably don't think about sexuality the same way at all.
I don't think attraction is that malleable.
Certainly my intuition based on day to day experience and observations is the same, and alleged very large cultural differences are puzzling to me and I wouldn't be surprised if the matter were resolved in your favor.
But note that if nothing else, the example shows that cultures vary in what they consider to be obviously unattractive.
But note that if nothing else, the example shows that cultures vary in what they consider to be obviously unattractive.
That's not necessarily true - we don't need to look too far from our own culture to see intentional downplaying of attractiveness (modesty, "evil eye", etc)
I was gonna write more on this topic but then decided to just go and check what the anthropologists report the Mursi themselves say concerning lip plates:
http://www.mursi.org/pdf/latosky.pdf
Even after reading, it's still not quite clear.
My interpretation of this is that it's less about sheer beauty and more a way of being what in our culture we'd call "put together". A woman who does not wear her plate during the ritual periods when being put together is necessary is perceived as what we'd call "sloppy", and it would be associated with a lack of discipline in other areas of life. (It's also a tribal identity marker and a way to make money from tourists, of course)
The plate definitely maps onto something which is present in our own psychology, but I'm not at all convinced that it's attractiveness. I think you're right that it doesn't actively ruin attractiveness, which does indicate malleability.
(By the way, historically the anthropologists first thought it was an anti-rape measure (but the Mursi denied it), and then they thought it was a beauty mark which determined higher dowry (until it was discovered that dowry was set at birth). That second misconception is probably why it circulates on the internet as an example of divergent beauty standards. That's not to say that it isn't divergent beauty standards, but it's not just that.)
Day-to-day experience and observation can give you evidence that attraction isn't very malleable in adults. Do your experience and observation tell you anything about whether, e.g., who you end up being attracted to depends strongly on the people you see around you before age 5?
Related:
Is Kindness Physically Attractive?
The tl;dr version: The halo effect of beauty also works in reverse: if you start liking a person, they'll start to appear more physically attractive, as well.
Thanks for pointing out the article. My findings are in tension with the ones that the article reports on: I have an instrumental variables type argument based on the data in the opposite direction (which I'll write about soon), and I think the the phenomena in the speed dating data is more likely to generalize because it was collected in a real world setting rather than a lab.
On the other hand, anecdotally, it seems like the situation may be very different if one looks at perceptions based on interactions that occur over longer time horizons than 4 minute speed dates.
FYI, you have a sentence missing its end: “The standard deviations of the distributions are nearly identical: 0.6 points on a ”.
Also, I think the "refined estimate" plot would be improved by setting its x-axis scale to be the same as the previous plot (even though this would create empty space).
*There is a universal standard for beauty.
*Beauty is in the eye of the beholder.
Just putting this out there - beauty is in fact completely subjective, and there is no universal standard nor can there be one, HOWEVER, it seems to us like beauty is objective because humans are really genetically (and socially) similar to each other. This gives rise to preferences that are shared by large groups, and the illusion that the things which many people consider attractive are objectively beautiful.
Yes, I meant "subjective" in a colloquial sense (the way people use it in day to day conversation) rather than a philosophical sense.
It seems possible to me that there are standards of beauty that would cut across many different species of intelligent life (including extraterrestrials) out of virtue of there being similar evolutionary pressures across contexts: for example, I could imagine aliens typically viewing aliens with symmetric features as being more attractive than aliens with asymmetric features. But yes, it's in principal possible for an entity's conceptions of beauty to be completely orthogonal to those of humans.
Has anyone investigated this in non-human animals here on earth?
(... I realise that I have no idea how commonly, and how strongly, visual "attractiveness" is relevant to mating of non-human animals at all. Clearly at least sometimes it's at least quite relevant (consider, e.g., peacocks), but beyond that I'm pretty clueless. If you're reading this and know much more, please educate me!)