Birth order effect found in Nobel Laureates in Physics
28Unnamed
6Bucky
18Unnamed
4Eli Tyre
2Leafcraft
0Pattern
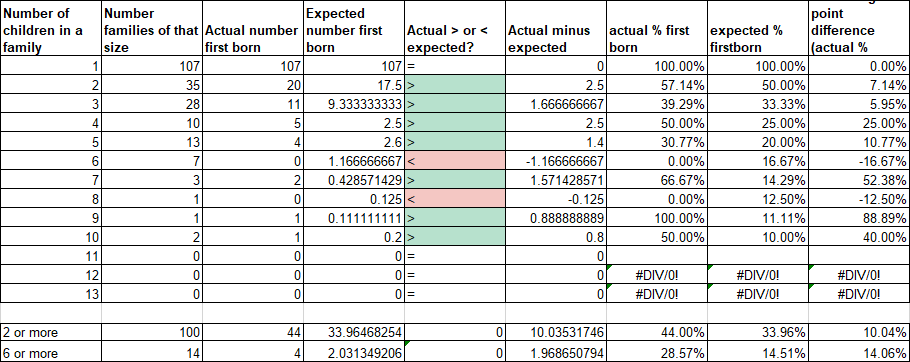
10tenthkrige
1Bucky
1tenthkrige
1Pattern
3Mary Wang
16Bucky
11Eli Tyre
6Ben Pace
4DanielFilan
3jmh
1Bucky
1TheMajor
3Bucky
2TheMajor
0Pattern
2jmh
0Pattern
1jmh
0Pattern
New Comment
Now that we have data on LWers/SSCers, mathematicians, and physicists, if anyone wants to put more work into this I'd like to see them look someplace different. We don't want to fall into the Wason 2-4-6 trap of only looking for birth order effects among smart STEM folks. We want data that can distinguish Scott's intelligence / intellectual curiosity hypothesis from other possibilities like some non-big-5 personality difference or a general firstborns more likely phenomenon.
Very good point. That would mean examining Nobel laureates in chemistry, medicine and economics might not give much extra information.
Literature laureates would, I presume, have significant intellectual curiosity so might give an indication as to whether the effect is specific to STEM subjects or more generalised curiosity/something else.
I’m not sure about Peace laureates – a negative result wouldn’t tell us much but a positive result would be interesting.
Any other suggestions for data which might be publicly available and point us towards one hypothesis or another?
We’re only going to get a good picture of what’s going on by someone deciding to do a proper research project on this but I feel like any additional data which people could collect would be helpful to point the project in the right direction.
Seems interesting to get data on:
Some group that isn't heavily selected for intelligence / intellectual curiosity: skateboarders, protestors, professional hockey players...
Some non-STEM group that is selected for success based on mental skills: literature laureates, governors, ...
Not sure which groups it would be easy to get data on.
There is also the option of looking into existing research on birth order to see what groups other people have already looked at.
I'm interested in teasing apart "high achievement" from "high achievement in a STEM field".
I'd be interested in analysis of fortune 500 CEOs, for instance.
The suggestion about Fortune 500 CEO seems good; "self-made" millionaires are a category far enough from STEM, and, due to their status, they are more likely to have reliable biographical information. If you want to go in a completely different direction, how about something like the Darwin Awards?
Staying 'out of the 2-4-6' does seem useful, but it'd also be nice to know if it is a 'STEM thing'.
Which you also can't know if you don't test other fields. I think there are at least 3 concentric levels to distinguish : ( famous ( intelligent ( STEM ) ) ).
So potentially ( Sports players ( Literature laureates / governors ( Physicists / Mathematicians ) ) ) ?
Some sports players are pretty smart and probably some governors aren't. What about ( Reality TV celebrities ( heads of state of UNSC countries ( Physicists / Mathematicians / Engineers ) ) ).
(1 minute of thought did not provide another group of famous & not-even-a-little-bit-selected-for-intelligence people, unless there's a database of lottery winners, which I doubt. Curious for suggestions.)
(Famous engineers: of course Wikipedia does not disappoint.)
That's a good point, which applies to both this and the prior post. The reason 'Nobel' Laureates are easy is probably the fame component.
Olympic medalists? Not just intelligence but a certain kind of political savvy and perseverance.
This is a review of my own post.
The first thing to say is that for the 2018 Review Eli’s mathematicians post should take precedence because it was him who took up the challenge in the first place and inspired my post. I hope to find time to write a review on his post.
If people were interested (and Eli was ok with it) I would be happy to write a short summary of my findings to add as a footnote to Eli’s post if it was chosen for the review.
***
This was my first post on LessWrong and looking back at it I think it still holds up fairly well.
There are a couple of things I would change if I were doing it again:
- Put less time into the sons vs daughters thing. I think this section could have two thirds of it chopped out without losing much.
- Unnamed’s comment is really important in pointing out a mistake I was making in my final paragraph.
- I might have tried to analyse whether it is a firstborn thing vs an earlyborn thing. In the SSC data it is strongly a firstborn thing and if I combined Eli and my datasets I might be able to confirm whether this is also the case in our datasets. I’m not sure if this would provide a decisive answer as our sample size is much smaller even when combining the sets.
Thanks for doing this!
I feel more validated in having spent the time doing data-collection for the mathematician data set after seeing that it prompted someone else to investigate in this area. It's pretty encouraging to know that if I write up something of interest on LessWrong, other people might build on it.
This feels like a pretty central example of 'things we found out on lesswrong in 2018'. Great work all round, so I'm nominating it. Next year, I'll also nominate the further work on this that came out in 2019.
This is now a hypothesis I look out for and see many places, thanks in part to this post.
Just asking about the birth order here. What is the implication of the finding -- why is this seen? Any thoughts?
One of the things I find most interesting is that the effect seems to be strongest for the rationality community.
I would suggest a sister theory to the intellectual curiosity angle that Scott mentioned. Eldest children spend their formative years without another child in the family to look up to. I would think that this would lead to, on average, a lower acceptance of information from authority.
This comes a bit my personal experience - I had an elder brother whose opinion I would take on as my own, even through my teens. I'd be interested to know if other younger siblings in the community have had a similar experience.
It would explain why the effect is so strong in the rationalist community. In Science and Maths it helps to challenge authoritative sources but this is actively encouraged, so the intrinsic effect needs to be less strong to get you to do it. The rationalist community is often challenging things which, culturally, aren't supposed to be challenged which would give a higher intrinsic bar to entry.
I would suggest Regression to the Mean instead - we are only interested in this hypothesis because of its unusual high number on the survey in the first place.
I wandered about regression to the mean but with the SSC data being so large there isn’t much room for a big effect - even moving 4SD on the SSC data won’t move the mean much. I’m afraid I don’t know how to do the maths beyond comparing confidence intervals as I did in the text.
You're right, I should have double-checked the standard deviations before suggesting regression to the mean. I agree that regression doesn't plausibly explain the data.
Just wild guesses.
I had a question about how adoption fits in to the picture, so two things come to mind:
A) It still exists for adopted kids.
Since it's not clear what the effect seems meaningless for single children, maybe it doesn't exist for them.
Maybe older siblings are trusted with more responsibility or specifically watching out for younger siblings, and that has way more effect than anyone expected. This would be a pain to test, but one might try to check by seeing if the gaps between the ages of kids matter. It's a pretty specific hypothesis though, so maybe it'd be something else about interacting a lot with younger kids while young (but older).
B) It doesn't.
That might mean it has something to do with birth, as opposed to differences in how later/earlier children are raised.
I did a search on the first born more intelligent query and go a hit to some article published in late 2016 or early 2017 -- news paper reported on the study in Feb 2017. What the hypothesis seemed to be was that parent interact with the first child differently than the later children and provide a more mentally stimulating environment for that child.
If so any bets on when the first law suit for compensation by the younger siblings will be filed for a great share of any inheritance? (semi-joking...)
I was reading through the comments on "Advances in baby formula" and I noticed 2 claims: babies that are breastfed have higher IQ, and mothers breastfeed less with later children.
If so I wonder if that might not be traced back to immune systems -- breastfeeding allows the baby to develop a strong immune system I think given the baby can borrow from mom rather than developing the response alone.